 Backend Development
Backend Development
 C++
C++
 What is the area of the largest possible rhombus that can be inscribed in a rectangle?
What is the area of the largest possible rhombus that can be inscribed in a rectangle?
What is the area of the largest possible rhombus that can be inscribed in a rectangle?

The rhombus inscribed in a rectangle is tangent to the sides of the rectangle, so we can infer that the diagonal of the largest inscribed rhombus is equal to the length and width of the rectangle.
If we have the length (l) and width (b) of the rectangle, the diagonal lengths of the largest inscribed rhombus are d1 = l and d2 = b.
The area of a rhombus is given by the formula,
Area = (d1*d2)/2
Substituting the values of d1 and d2, we get:
Area = (l*b)/2
Using this formula, we can create a calculation Program that can inscribe the area of the largest rhombus within a rectangle,
Example
Real-time demonstration
#include <stdio.h>
int main() {
float l = 16, b = 6;
float area = (l*b)/2;
printf("The area of rhombus inscribed in a rectangle of length %f and breadth %f is %f", l,b,area);
return 0;
}Output
The area of rhombus inscribed in a rectangle of length 15 and breadth 12 is 90.
The above is the detailed content of What is the area of the largest possible rhombus that can be inscribed in a rectangle?. For more information, please follow other related articles on the PHP Chinese website!

Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

Video Face Swap
Swap faces in any video effortlessly with our completely free AI face swap tool!

Hot Article

Hot Tools

Notepad++7.3.1
Easy-to-use and free code editor

SublimeText3 Chinese version
Chinese version, very easy to use

Zend Studio 13.0.1
Powerful PHP integrated development environment

Dreamweaver CS6
Visual web development tools

SublimeText3 Mac version
God-level code editing software (SublimeText3)

Hot Topics
 1666
1666
 14
14
 1425
1425
 52
52
 1325
1325
 25
25
 1272
1272
 29
29
 1252
1252
 24
24
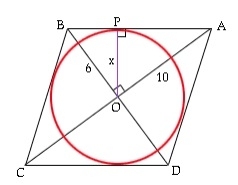 What is the area of a circle inscribed in a rhombus?
Sep 05, 2023 am 08:25 AM
What is the area of a circle inscribed in a rhombus?
Sep 05, 2023 am 08:25 AM
The inscribed circle of a rhombus is tangent to its four sides and four endpoints. The sides of the rhombus are tangent to the circle. Here, r is the radius found using a and the diagonal of the given value. Now the area triangle AOB = ½*OA*OB = ½*AB*r (both using the formula ½*b*h). ½*a/2*b/2=½*(√(a2/4+b2/4))*ra*b/8=√(a2+b2)*r/4r=a*b/2√(a2 +b2) Circle area = π*r*r=π*(a2*b2)/4(a2support>+b2) Example of the diagonals of rhombus 5 and 10. Area is 15.700000 Example code Real-time demonstration #include<stdio.h>intma
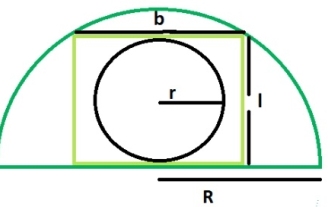 What is the area of a circle within a rectangle inscribed in a semicircle?
Sep 13, 2023 am 08:45 AM
What is the area of a circle within a rectangle inscribed in a semicircle?
Sep 13, 2023 am 08:45 AM
A circle inscribed in a rectangle is tangent to the longer side of the rectangle, that is, its length is tangent to the circle. A rectangle inscribed in a semicircle touches two points on the arc of the semicircle. The width of the rectangle is equal to the diameter of the circle. If R is the radius of the semicircle. The length of the rectangle = √2R/2 The width of the rectangle = R/√2 The radius of the inscribed circle is r = b/2 = R/2√2 Using this formula we can calculate the rectangle inscribed in the semicircle The area of a circle, area = (π*r2)=π*R/8 Example Demonstration #include<stdio.h>intmain(){&
 Write a program in C language to print solid and hollow diamond patterns
Aug 29, 2023 am 09:33 AM
Write a program in C language to print solid and hollow diamond patterns
Aug 29, 2023 am 09:33 AM
Program Description Print the pattern of solid and hollow rhombus as shown below
 How to merge a graphic after CAD rectangles are scattered
Feb 28, 2024 pm 12:10 PM
How to merge a graphic after CAD rectangles are scattered
Feb 28, 2024 pm 12:10 PM
When using CAD software, we often encounter situations where we need to recombine "scattered" rectangular objects into a single graphic. This need arises in many fields, such as space planning, mechanical design and architectural drawings. In order to meet this demand, we need to understand and master some key functions in CAD software. Next, the editor of this website will introduce you in detail how to complete this task in the CAD environment. Users who have doubts can come and follow this article to learn. Method for merging CAD rectangles into one graphic after breaking them up: 1. Open the CAD2023 software, create a rectangle, and then enter the X command and a space. As shown below: 2. Select the rectangular object and space it. You can break up the objects. 3. Select all open lines
 Find the area of a circle inscribed in a square in Java
Aug 19, 2023 pm 04:53 PM
Find the area of a circle inscribed in a square in Java
Aug 19, 2023 pm 04:53 PM
Acircleisaroundshapetwo-dimensionaldiagramwhichhasnocorners.Everycirclehasanoriginpointandeverypointonthecirclemaintainsequaldistancefromtheorigin.ThedistancebetweentheoriginandapointinacircleisknownasRadiusofthecircle.Andsimilarly,ifwedrawalinefromo
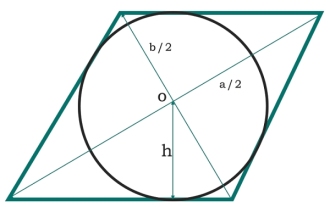 In the C program, what is the area of a circle inscribed in a rhombus?
Sep 01, 2023 pm 09:29 PM
In the C program, what is the area of a circle inscribed in a rhombus?
Sep 01, 2023 pm 09:29 PM
Here we will see the area of a circle inscribed in a rhombus. The diagonals of the rhombus are 'a' and 'b' respectively. The radius of the circle is h. Two diagonals form four equal triangles. Every triangle is a right triangle, so their area is - Each side of the rhombus is the hypotenuse - Therefore, the area of the circle is - Example#include<iostream>#include<cmath>usingnamespacestd;floatarea(floata,floatb) { if(a<0||b<0)//ifthevaluesaren
 How to print stars in a diamond pattern using C language?
Sep 03, 2023 pm 02:41 PM
How to print stars in a diamond pattern using C language?
Sep 03, 2023 pm 02:41 PM
Here, to print stars in a diamond pattern, we use nested for loops. The logic we use to print stars in a diamond pattern is as follows - //Forupperhalfofthediamondthelogicis:for(j=1;j<=rows;j++){ for(i=1;i<=rows-j;i++) printf(" "); for(i=1;i<=2*j-1;i++
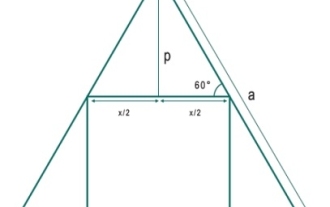 What is the largest square that can be inscribed in an equilateral triangle?
Sep 22, 2023 pm 06:21 PM
What is the largest square that can be inscribed in an equilateral triangle?
Sep 22, 2023 pm 06:21 PM
Here we will see the area of the largest square that can be inscribed in an equilateral triangle. The side length of the triangle is 'a' and the side length of the square is x. The side length 'a' of the triangle is − so x is − Example #include<iostream>#include<cmath>usingnamespacestd;floatareaSquare(floata){//aissideoftriangle if(a<0)//ifaisnegative,thenthisisi



