How to build a binary tree using java
Directory:
1. Put a Assign the value of array to a binary tree
2. Specific code
Note:
1. The subscript of the parent node array ranges from 0 to n/2 -1, but it should be less than n/2-1 when traversing, because the last The parent node may not have a right child. When n/2-1 is an odd number, it has a right child, and when it is an even number, it only has a left child.
##2. Node. The subscript of the left child is 2n+1, and the subscript of the right child is 2n+2
##1. Tree construction method 
2. Specific code
package tree;
import java.util.LinkedList;
import java.util.List;
/**
* 功能:把一个数组的值存入二叉树中,然后进行3种方式的遍历
*
* 参考资料0:数据结构(C语言版)严蔚敏
*
* 参考资料1:http://zhidao.baidu.com/question/81938912.html
*
* 参考资料2:http://cslibrary.stanford.edu/110/BinaryTrees.html#java
*
* @author ocaicai@yeah.net @date: 2011-5-17
*
*/
public class BinTreeTraverse2 {
private int[] array = { 1, 2, 3, 4, 5, 6, 7, 8, 9 };
private static List<Node> nodeList = null;
/**
* 内部类:节点
*
* @author ocaicai@yeah.net @date: 2011-5-17
*
*/
private static class Node {
Node leftChild;
Node rightChild;
int data;
Node(int newData) {
leftChild = null;
rightChild = null;
data = newData;
}
}
public void createBinTree() {
nodeList = new LinkedList<Node>();
// 将一个数组的值依次转换为Node节点
for (int nodeIndex = 0; nodeIndex < array.length; nodeIndex++) {
nodeList.add(new Node(array[nodeIndex]));
}
// 对前lastParentIndex-1个父节点按照父节点与孩子节点的数字关系建立二叉树
for (int parentIndex = 0; parentIndex < array.length / 2 - 1; parentIndex++) {
// 左孩子
nodeList.get(parentIndex).leftChild = nodeList
.get(parentIndex * 2 + 1);
// 右孩子
nodeList.get(parentIndex).rightChild = nodeList
.get(parentIndex * 2 + 2);
}
// 最后一个父节点:因为最后一个父节点可能没有右孩子,所以单独拿出来处理
int lastParentIndex = array.length / 2 - 1;
// 左孩子
nodeList.get(lastParentIndex).leftChild = nodeList
.get(lastParentIndex * 2 + 1);
// 右孩子,如果数组的长度为奇数才建立右孩子
if (array.length % 2 == 1) {
nodeList.get(lastParentIndex).rightChild = nodeList
.get(lastParentIndex * 2 + 2);
}
}
/**
* 先序遍历
*
* 这三种不同的遍历结构都是一样的,只是先后顺序不一样而已
*
* @param node
* 遍历的节点
*/
public static void preOrderTraverse(Node node) {
if (node == null)
return;
System.out.print(node.data + " ");
preOrderTraverse(node.leftChild);
preOrderTraverse(node.rightChild);
}
/**
* 中序遍历
*
* 这三种不同的遍历结构都是一样的,只是先后顺序不一样而已
*
* @param node
* 遍历的节点
*/
public static void inOrderTraverse(Node node) {
if (node == null)
return;
inOrderTraverse(node.leftChild);
System.out.print(node.data + " ");
inOrderTraverse(node.rightChild);
}
/**
* 后序遍历
*
* 这三种不同的遍历结构都是一样的,只是先后顺序不一样而已
*
* @param node
* 遍历的节点
*/
public static void postOrderTraverse(Node node) {
if (node == null)
return;
postOrderTraverse(node.leftChild);
postOrderTraverse(node.rightChild);
System.out.print(node.data + " ");
}
public static void main(String[] args) {
BinTreeTraverse2 binTree = new BinTreeTraverse2();
binTree.createBinTree();
// nodeList中第0个索引处的值即为根节点
Node root = nodeList.get(0);
System.out.println("先序遍历:");
preOrderTraverse(root);
System.out.println();
System.out.println("中序遍历:");
inOrderTraverse(root);
System.out.println();
System.out.println("后序遍历:");
postOrderTraverse(root);
}
}Output result:
先序遍历: 1 2 4 8 9 5 3 6 7 中序遍历: 8 4 9 2 5 1 6 3 7 后序遍历: 8 9 4 5 2 6 7 3 1
The above is the detailed content of How to build a binary tree using java. For more information, please follow other related articles on the PHP Chinese website!

Hot AI Tools

Undresser.AI Undress
AI-powered app for creating realistic nude photos

AI Clothes Remover
Online AI tool for removing clothes from photos.

Undress AI Tool
Undress images for free

Clothoff.io
AI clothes remover

Video Face Swap
Swap faces in any video effortlessly with our completely free AI face swap tool!

Hot Article

Hot Tools

Notepad++7.3.1
Easy-to-use and free code editor

SublimeText3 Chinese version
Chinese version, very easy to use

Zend Studio 13.0.1
Powerful PHP integrated development environment

Dreamweaver CS6
Visual web development tools

SublimeText3 Mac version
God-level code editing software (SublimeText3)

Hot Topics
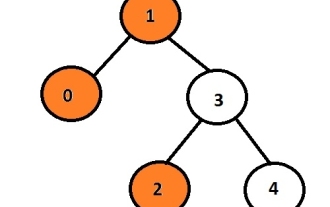 Print left view of binary tree in C language
Sep 03, 2023 pm 01:25 PM
Print left view of binary tree in C language
Sep 03, 2023 pm 01:25 PM
The task is to print the left node of the given binary tree. First, the user will insert data, thus generating a binary tree, and then print the left view of the resulting tree. Each node can have at most 2 child nodes so this program must iterate over only the left pointer associated with the node if the left pointer is not null it means it will have some data or pointer associated with it otherwise it will be printed and displayed as the left child of the output. ExampleInput:10324Output:102Here, the orange node represents the left view of the binary tree. In the given graph the node with data 1 is the root node so it will be printed and instead of going to the left child it will print 0 and then it will go to 3 and print its left child which is 2 . We can use recursive method to store the level of node
 Detailed explanation of binary tree structure in Java
Jun 16, 2023 am 08:58 AM
Detailed explanation of binary tree structure in Java
Jun 16, 2023 am 08:58 AM
Binary trees are a common data structure in computer science and a commonly used data structure in Java programming. This article will introduce the binary tree structure in Java in detail. 1. What is a binary tree? In computer science, a binary tree is a tree structure in which each node has at most two child nodes. Among them, the left child node is smaller than the parent node, and the right child node is larger than the parent node. In Java programming, binary trees are commonly used to represent sorting, searching and improving the efficiency of data query. 2. Binary tree implementation in Java In Java, binary tree
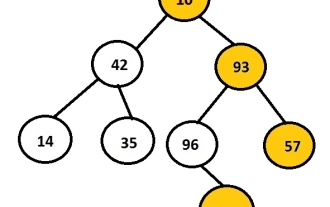 In C language, print the right view of the binary tree
Sep 16, 2023 pm 11:13 PM
In C language, print the right view of the binary tree
Sep 16, 2023 pm 11:13 PM
The task is to print the right node of the given binary tree. First the user will insert data to create a binary tree and then print a right view of the resulting tree. The image above shows a binary tree created using nodes 10, 42, 93, 14, 35, 96, 57 and 88, with the nodes on the right side of the tree selected and displayed. For example, 10, 93, 57, and 88 are the rightmost nodes of the binary tree. Example Input:1042931435965788Output:10935788 Each node has two pointers, the left pointer and the right pointer. According to this question, the program only needs to traverse the right node. Therefore, the left child of the node does not need to be considered. The right view stores all nodes that are the last node in their hierarchy. Therefore, we can
 How to implement binary tree traversal using Python
Jun 09, 2023 pm 09:12 PM
How to implement binary tree traversal using Python
Jun 09, 2023 pm 09:12 PM
As a commonly used data structure, binary trees are often used to store data, search and sort. Traversing a binary tree is one of the very common operations. As a simple and easy-to-use programming language, Python has many methods to implement binary tree traversal. This article will introduce how to use Python to implement pre-order, in-order and post-order traversal of a binary tree. Basics of Binary Trees Before learning how to traverse a binary tree, we need to understand the basic concepts of a binary tree. A binary tree consists of nodes, each node has a value and two child nodes (left child node and right child node
 The number of isosceles triangles in a binary tree
Sep 05, 2023 am 09:41 AM
The number of isosceles triangles in a binary tree
Sep 05, 2023 am 09:41 AM
A binary tree is a data structure in which each node can have up to two child nodes. These children are called left children and right children respectively. Suppose we are given a parent array representation, you have to use it to create a binary tree. A binary tree may have several isosceles triangles. We have to find the total number of possible isosceles triangles in this binary tree. In this article, we will explore several techniques for solving this problem in C++. Understanding the problem gives you a parent array. You have to represent it in the form of a binary tree so that the array index forms the value of the tree node and the value in the array gives the parent node of that particular index. Note that -1 is always the root parent. Given below is an array and its binary tree representation. Parentarray=[0,-1,3,1,
 Detailed explanation of Java binary tree implementation and specific application cases
Jun 15, 2023 pm 11:03 PM
Detailed explanation of Java binary tree implementation and specific application cases
Jun 15, 2023 pm 11:03 PM
Detailed explanation of Java binary tree implementation and specific application cases. Binary tree is a data structure often used in computer science and can perform very efficient search and sort operations. In this article, we will discuss how to implement a binary tree in Java and some of its specific application cases. Definition of Binary Tree Binary tree is a very important data structure, consisting of the root node (the top node of the tree) and several left subtrees and right subtrees. Each node has at most two child nodes, the child node on the left is called the left subtree, and the child node on the right is called the right subtree. If the node does not have
 Binary tree algorithm in PHP and FAQs
Jun 09, 2023 am 09:33 AM
Binary tree algorithm in PHP and FAQs
Jun 09, 2023 am 09:33 AM
With the continuous development of web development, PHP, as a widely used server scripting language, its algorithms and data structures are becoming more and more important. Among these algorithms and data structures, the binary tree algorithm is a very important concept. This article will introduce the binary tree algorithm and its applications in PHP, as well as answers to common questions. What is a binary tree? A binary tree is a tree structure in which each node has at most two child nodes, a left child node and a right child node. If a node has no child nodes, it is called a leaf node. Binary trees are often used for searching
 Methods and applications of binary tree implementation in PHP
Jun 18, 2023 pm 06:28 PM
Methods and applications of binary tree implementation in PHP
Jun 18, 2023 pm 06:28 PM
In computer science, a binary tree is an important data structure. It consists of nodes and the edges pointing to them, with each node connecting up to two child nodes. Binary trees are widely used in fields such as search algorithms, compilers, databases, and memory management. Many programming languages support the implementation of binary tree data structures, PHP being one of them. This article will introduce how PHP implements binary trees and its applications. Definition of Binary Tree A binary tree is a data structure that consists of nodes and edges pointing to them. Each node is connected to at most two child nodes,






