資料結構與演算法：圖

介紹

圖是一種資料結構，具有多個頂點（節點）和它們之間的邊（連接）。

樹是圖的一個例子。每棵樹都是一個圖，但並非每個圖都是樹，例如，有環的圖就不是樹。一棵樹將有一個根和兩個節點之間的一條唯一路徑，而圖可以有多個根和頂點之間的多個路徑。

基本術語

頂點: 圖中的節點。

邊: 兩個頂點之間的連接。

有向: 當兩個頂點之間的連接有方向時。這意味著只有一種方法可以從一個頂點到達另一個頂點。一個例子是顯示城市（頂點）和它們之間的路線（邊）的圖表。

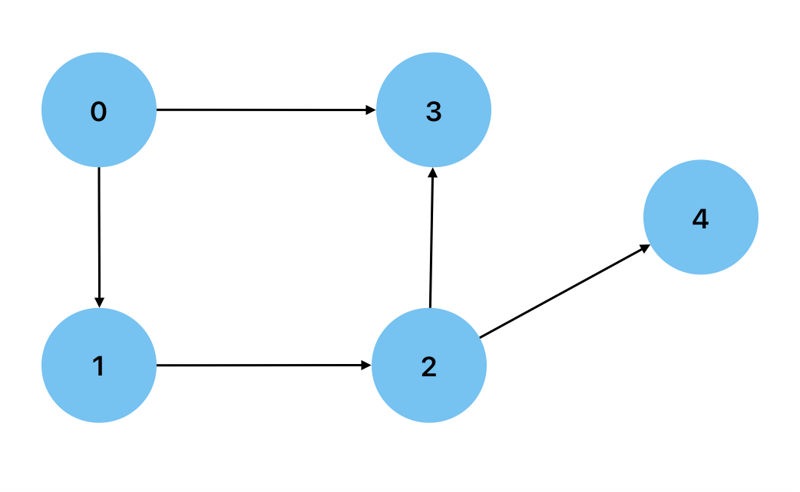
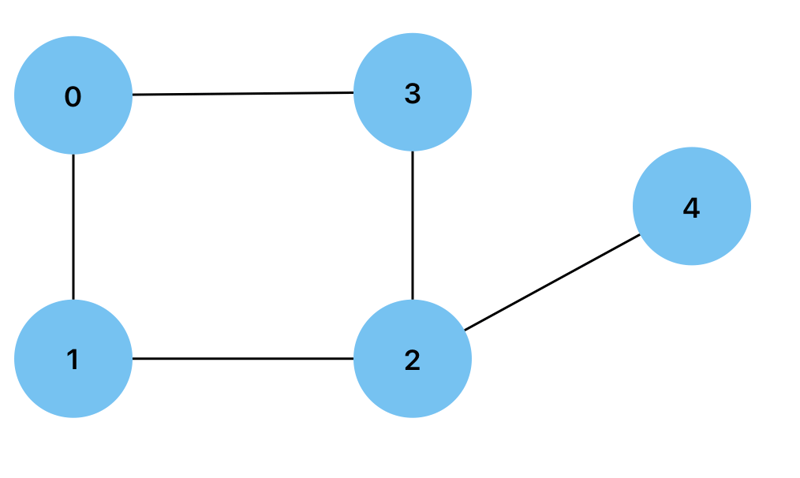
無向: 當圖上兩個頂點之間的連接是雙向的。一個例子是顯示透過友誼連結的人（頂點）的圖表。

度數: 連接到頂點的邊數。有向圖的頂點可以有入度或出度，分別是指向和遠離頂點的邊的數量。

加權: 邊的值作為權重的圖。一個例子是路線圖，其中節點之間的距離表示為權重。

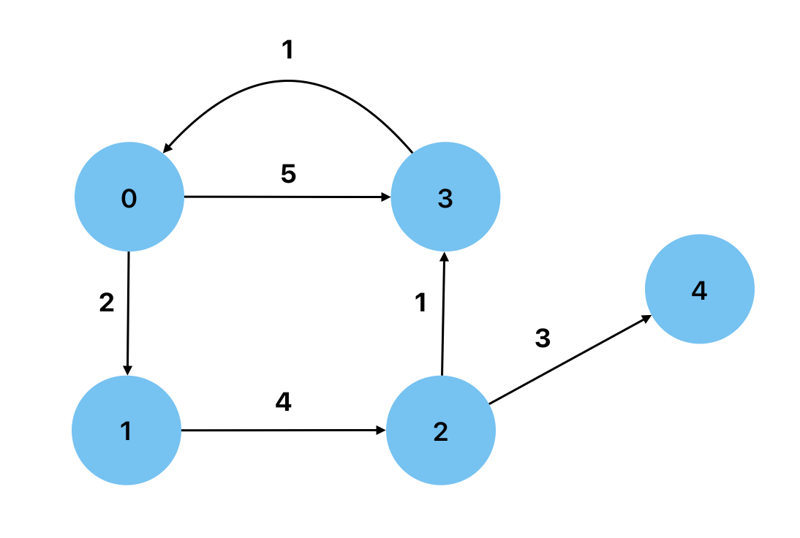
循環： 具有從至少一個頂點返回自身的路徑的圖。

非循環: 沒有循環的圖，也就是說，沒有節點有回自身的路徑。 有向無環圖是一種可用來顯示資料處理流程的圖。

密集:當圖的邊數接近最大可能數時

稀疏: 當圖的邊數接近最小可能數量時。

自循環: 當一條邊有一個頂點連結到其自身時。

多邊: 當圖在兩個頂點之間具有多條邊時。

簡單:當圖沒有自環或多邊時。

取得簡單有向圖中的最大邊數：n*(n-1)，其中 n 是節點數。

要取得簡單無向圖中的最大邊數：n*(n-1)/2，其中 n 是節點數。

在 JavaScript 中實作圖表

要實現圖，我們可以從指定圖的頂點和邊開始，下面是如何在給定圖的情況下執行此操作的範例：

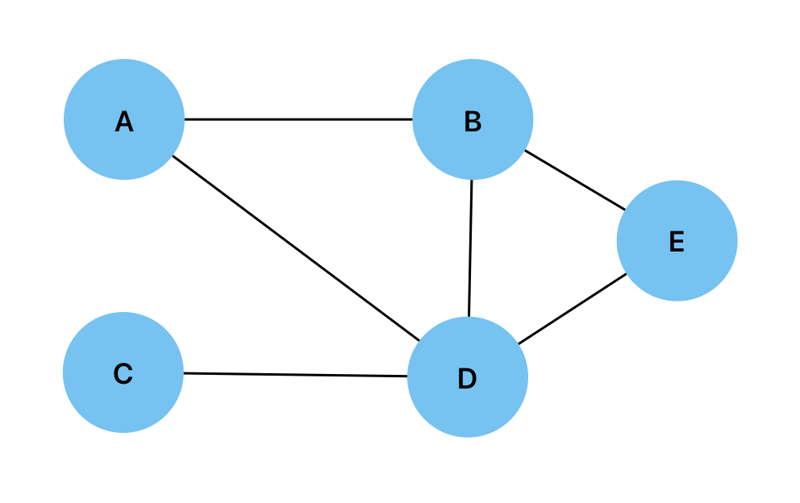
const vertices = ["A", "B", "C", "D", "E"];

const edges = [
  ["A", "B"],
  ["A", "D"],
  ["B", "D"],
  ["B", "E"],
  ["C", "D"],
  ["D", "E"],
];

然後我們可以建立一個函數來尋找與給定頂點相鄰的內容：

const findAdjacentNodes = function (node) {
  const adjacentNodes = [];
  for (let edge of edges) {
    const nodeIndex = edge.indexOf(node);
    if (nodeIndex > -1) {
      let adjacentNode = nodeIndex === 0 ? edge[1] : edge[0];
      adjacentNodes.push(adjacentNode);
    }
  }
  return adjacentNodes;
};

還有另一個檢查兩個頂點是否連接的函數：

const isConnected = function (node1, node2) {
  const adjacentNodes = new Set(findAdjacentNodes(node1));
  return adjacentNodes.has(node2);
};

鄰接表

鄰接清單是圖的表示形式，其中連接到節點的所有頂點都儲存為清單。下面是一個圖表及其對應鄰接清單的直觀表示。

鄰接列表可以透過建立兩個類別（Node 類別和 Graph 類別）在 JavaScript 中實現。 Node 類別將包含一個建構函式和一個連接兩個頂點的 connect() 方法。它還具有 isConnected() 和 getAdjacentNodes() 方法，其工作方式與上面所示的完全相同。

class Node {
  constructor(value) {
    this.value = value;
    this.edgesList = [];
  }
  connect(node) {
    this.edgesList.push(node);
    node.edgesList.push(this);
  }
  getAdjNodes() {
    return this.edgesList.map((edge) => edge.value);
  }
  isConnected(node) {
    return this.edgesList.map((edge) => 
    edge.value).indexOf(node.value) > -1;
  }
}

Graph 類別由建構子和 addToGraph() 方法組成，該方法會為圖中新增頂點。

class Graph {
  constructor(nodes) {
    this.nodes = [...nodes];
  }
  addToGraph(node) {
    this.nodes.push(node);
  }
}

Adjacency Matrix

A 2-D array where each array represents a vertex and each index represents a possible connection between vertices. An adjacency matrix is filled with 0s and 1s, with 1 representing a connection. The value at adjacencyMatrix[node1][node2] will show whether or not there is a connection between the two specified vertices. Below is is a graph and its visual representation as an adjacency matrix.

To implement this adjacency matrix in JavaScript, we start by creating two classes, the first being the Node class:

class Node {
  constructor(value) {
    this.value = value;
  }
}

We then create the Graph class which will contain the constructor for creating the 2-D array initialized with zeros.

class Graph {
  constructor(nodes) {
    this.nodes = [...nodes];
    this.adjacencyMatrix = Array.from({ length: nodes.length },   
    () => Array(nodes.length).fill(0));
   }
}

We will then add the addNode() method which will be used to add new vertices to the graph.

  addNode(node) {
    this.nodes.push(node);
    this.adjacencyMatrix.forEach((row) => row.push(0));
    this.adjacencyMatrix.push(new Array(this.nodes.length).fill(0));
  }

Next is the connect() method which will add an edge between two vertices.

  connect(node1, node2) {
    const index1 = this.nodes.indexOf(node1);
    const index2 = this.nodes.indexOf(node2);

    if (index1 > -1 && index2 > -1) {
      this.adjacencyMatrix[index1][index2] = 1;
      this.adjacencyMatrix[index2][index1] = 1; 
    }
  }

We will also create the isConnected() method which can be used to check if two vertices are connected.

  isConnected(node1, node2) {
    const index1 = this.nodes.indexOf(node1);
    const index2 = this.nodes.indexOf(node2);

    if (index1 > -1 && index2 > -1) {
      return this.adjacencyMatrix[index1][index2] === 1;
    }
    return false;
  }

Lastly we will add the printAdjacencyMatrix() method to the Graph class.

  printAdjacencyMatrix() {
    console.log(this.adjacencyMatrix);
  }

Breadth First Search

Similar to a Breadth First Search in a tree, the vertices adjacent to the current vertex are visited before visiting any subsequent children. A queue is the data structure of choice when performing a Breadth First Search on a graph.

Below is a graph of international airports and their connections and we will use a Breadth First Search to find the shortest route(path) between two airports(vertices).

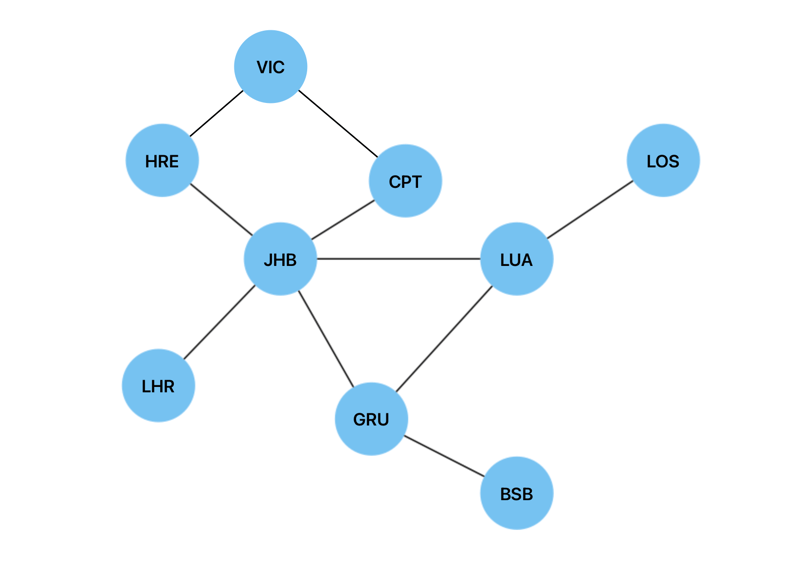
In order to implement this search algorithm in JavaScript, we will use the same Node and Graph classes we implemented the adjacency list above. We will create a breadthFirstTraversal() method and add it to the Graph class in order to traverse between two given vertices. This method will have the visitedNodes object, which will be used to store the visited vertices and their predecessors. It is initiated as null to show that the first vertex in our search has no predecessors.

breathFirstTraversal(start, end) {
    const queue = [start];
    const visitedNodes = {};
    visitedNodes[start.value] = null;

    while (queue.length > 0) {
      const node = queue.shift();

      if (node.value === end.value) {
        return this.reconstructedPath(visitedNodes, end);
      }
      for (const adjacency of node.edgesList) {
        if (!visitedNodes.hasOwnProperty(adjacency.value)) {
          visitedNodes[adjacency.value] = node;
          queue.push(adjacency);
        }
      }
    }
  }

Once the end vertex is found, we will use the reconstructedPath() method in the Graph class in order to return the shortest path between two vertices. Each vertex is added iteratively to the shortestPath array, which in turn must be reversed in order to come up with the correct order for the shortest path.

reconstructedPath(visitedNodes, endNode) {
    let currNode = endNode;

    const shortestPath = [];

    while (currNode !== null) {
      shortestPath.push(currNode.value);
      currNode = visitedNodes[currNode.value];
    }
    return shortestPath.reverse();
  }

In the case of the graph of international airports, breathFirstTraversal(JHB, LOS) will return JHB -> LUA -> LOS as the shortest path. In the case of a weighted graph, we would use Dijkstra's algorithm to find the shortest path.

Depth First Search

Similar to a depth first search in a tree, this algorithm will fully explore every descendant of a vertex, before backtracking to the root. A stack is the data structure of choice for depth first traversals in a graph.

A depth first search can be used to detect a cycle in a graph. We will use the same graph of international airports to illustrate this in JavaScript.

Similar to the Breadth First Search algorithm above, this implementation of a Depth First Search algorithm in JavaScript will use the previously created Node and Graph classes. We will create a helper function called depthFirstTraversal() and add it to the Graph class.

  depthFirstTraversal(start, visitedNodes = {}, parent = null) {
    visitedNodes[start.value] = true;

    for (const adjacency of start.edgesList) {
      if (!visitedNodes[adjacency.value]) {
        if (this.depthFirstTraversal(adjacency, visitedNodes, start)) {
          return true;
        }
      } else if (adjacency !== parent) {
        return true;
      }
    }

    return false;
  }

This will perform the Depth First Traversal of the graph, using the parent parameter to keep track of the previous vertex and prevent the detection of a cycle when revisiting the parent vertex. Visited vertices will be marked as true in the visitedNodes object. This method will then use recursion to visit previously unvisited vertices. If the vertex has already been visited, we check it against the parent parameter. A cycle has been found if the vertex has already been visited and it is not the parent.

We will also create the wrapper function hasCycle() in the Graph class. This function is used to detect a cycle in a disconnected graph. It will initialize the visitedNodes object and loop through all of the vertices in the graph.

hasCycle() {
    const visitedNodes = {};

    for (const node of this.nodes) {
      if (!visitedNodes[node.value]) {
        if (this.depthFirstTraversal(node, visitedNodes)) {
          return true;
        }
      }
    }
    return false;
  }

In the case of the graph of international airports, the code will return true.

Depth First Traversal of a graph is also useful for pathfinding(not necessarily shortest path) and for solving mazes.

結論

データ構造とアルゴリズムの研究を進めるには、データ構造としてのグラフとそれに関連するアルゴリズムをしっかりと理解することが絶対に必要です。このシリーズのこれまでの投稿ほど初心者向けではありませんが、このガイドはデータ構造とアルゴリズムについての理解を深めるのに役立つはずです。

以上是資料結構與演算法：圖的詳細內容。更多資訊請關注PHP中文網其他相關文章！